scipy.special.

jn_zeros

scipy.special.jn_zeros(n, nt)

Compute zeros of integer-order Bessel functions Jn.

Compute nt zeros of the Bessel functions \(J_n(x)\) on the interval \((0, \infty)\). The zeros are returned in ascending order. Note that this interval excludes the zero at \(x = 0\) that exists for \(n > 0\).

Parameters:

nint

Order of Bessel function

ntint

Number of zeros to return

Returns:

ndarray

First nt zeros of the Bessel function.

See also

jv

Real-order Bessel functions of the first kind

jnp_zeros

Zeros of \(Jn'\)

References

[1]

Zhang, Shanjie and Jin, Jianming. “Computation of Special Functions”, John Wiley and Sons, 1996, chapter 5. https://people.sc.fsu.edu/~jburkardt/f77_src/special_functions/special_functions.html

Examples

Try it in your browser!

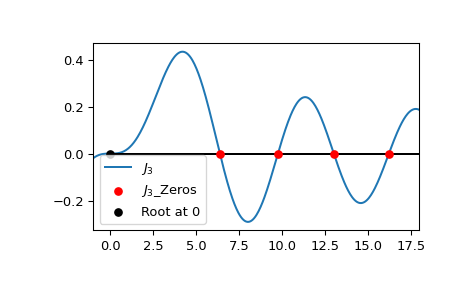
Compute the first four positive roots of \(J_3\).

>>> from scipy.special import jn_zeros
>>> jn_zeros(3, 4)
array([ 6.3801619 ,  9.76102313, 13.01520072, 16.22346616])

Plot \(J_3\) and its first four positive roots. Note that the root located at 0 is not returned by jn_zeros.

>>> import numpy as np
>>> import matplotlib.pyplot as plt
>>> from scipy.special import jn, jn_zeros
>>> j3_roots = jn_zeros(3, 4)
>>> xmax = 18
>>> xmin = -1
>>> x = np.linspace(xmin, xmax, 500)
>>> fig, ax = plt.subplots()
>>> ax.plot(x, jn(3, x), label=r'$J_3$')
>>> ax.scatter(j3_roots, np.zeros((4, )), s=30, c='r',
...            label=r"$J_3$_Zeros", zorder=5)
>>> ax.scatter(0, 0, s=30, c='k',
...            label=r"Root at 0", zorder=5)
>>> ax.hlines(0, 0, xmax, color='k')
>>> ax.set_xlim(xmin, xmax)
>>> plt.legend()
>>> plt.show()

Go BackOpen In Tab